Okay, I'm back from Tennessee. The talk went pretty well, and the conference was a lot of fun. So let's celebrate with another installment of Sunday Chess Problem!
Our last entry featured a series mover. It seemed to be well-received, and they happen to be fairly easy to blog. So let's try another one! The following problem was composed by Josif Krikheli in 1966. It calls for Series Helpmate in 25:
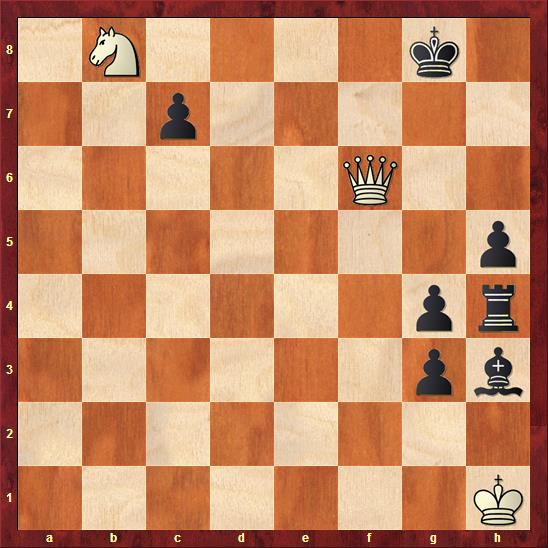
Our last entry called for Series Selfmate, so this one is a bit different. We have seen normal helpmates, in which black moves first and both sides cooperate to create a position in which black is checkmated, in no more than the required number of moves. In Series Helpmates, it is black who plays the series. Applied to our present problem, the idea is this: Black will play 25 consecutive moves. White will remain motionless during this time. After black completes the series, white will make one move and give checkmate to black's king. Black is free to capture any white pieces he chooses (except for the king, of course). However, at no point is he allowed to place himself in check, and at no point is he allowed to place white in check. Except for those two restrictions, it's all good!
Keep in mind that for a series mover to be considered sound, it must be that all of the moves have to be played in a specific order. If even two moves could be played in a different order, then the whole problem must be discarded as unsound. Part of the fun in these problems is working out why the moves must be played in one particular order.
Let's get down to business. Solving a problem such as this requires imagining what the final position could possibly be. White is only going to make one move, after all, and that move had better give checkmate. Our first thought might be to block in the black king in his present corner, but if you spend some time trying to make that work you will not be successful.
This makes us suspect a king march, but to where? With a little imagination, you might notice a5. If the black king is on a5, and some unit or other is blocking b4, then white will be able to give mate with Qa6.
Can we make that work? We can, but it requires some fancy footwork. In his trek to a5, the black king will have to cross the f-file, the h4-d8 diagonal, and the sixth rank. Since he must never pass through check, he will need to arrange for blockers. The bishop on h3 might be valuable for that purpose, but it is currently immobile (since it would discover check to the white king by moving). So, before the h3 bishop can join the party to provide a shield on f7, a preliminary shield on h2 must be arranged.
The outlines of the solution are becoming clear. Black begins by promoting his c-pawn: 1. c5 2. c4 3. c3 4. c2:
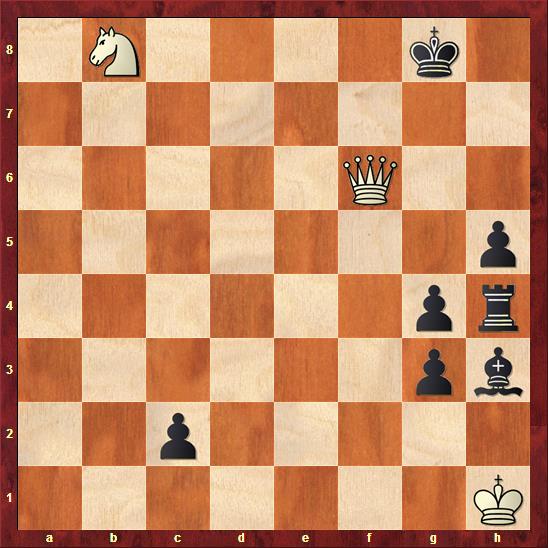
Perhaps we could simply have started with this pawn on c2, thereby making the problem a Series Helpmate in 21. On the other hand, maybe it's more dramatic to have the pawn march all the way down the board. Incidentally, a problem in which a pawn marches from its second rank all the way to promotion is said to exhibit the excelsior theme. Good to know!
Continuing: 5. c1B 6. Be3 7. Bg1 8. Bh2:
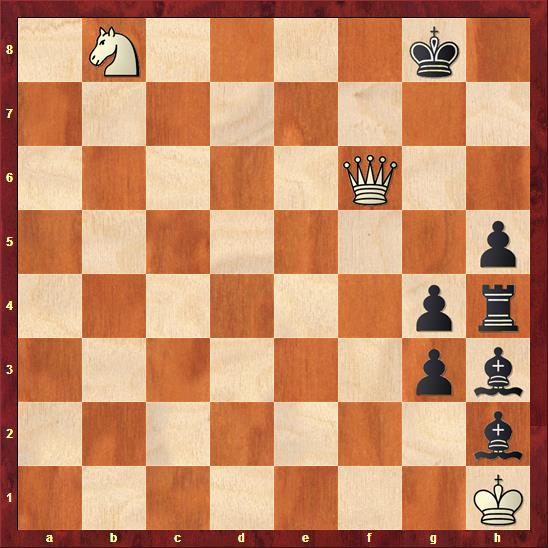
The first shield has been arranged. Now the h3B can provide a shield for its king: 9. Bf1 10. Bc4 11. Bf7:
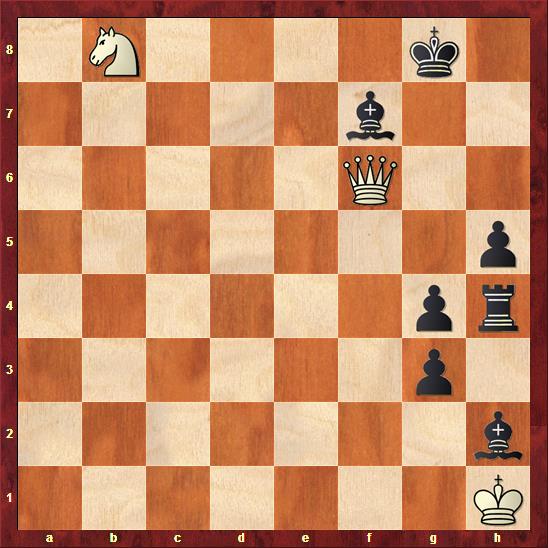
Now the king can begin its march: 12. Kf8 13. Ke8:
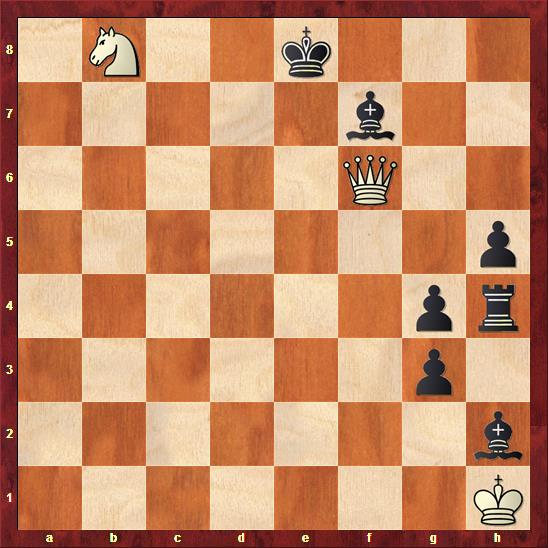
A good start, but the king has a long way to go. However, it is clear that the black king will require two further blocks to get to a5. Both of those blocks must occur on dark squares. So the light-squared bishop now returns to h3, to free up the dark-squared bishop for further king-escorting duties. The solution continues: 14. Bc4 15. Bf1 16. Bh3:
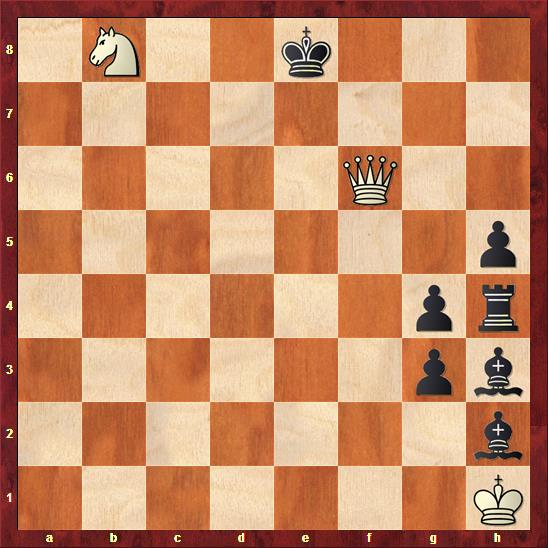
which frees up the dark-squared bishop to do this: 17. Bg1 18. Bc5 19. Be7:
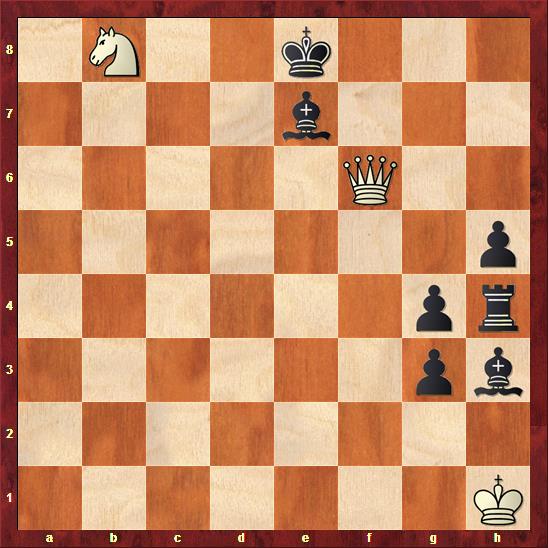
The king can now continue its journey: 20. Kd8 21. Kd7 22. Bd6:

The bishop provides one further block. The solution now concludes with 23. Kb6 24. Ka5 25. Bb4 Qa6 mate:

And there you go. Series movers are fun. See you next week!
- Log in to post comments

Again, quite pleasant. Particularly sweet start with the pawn moves & promotion. Then the blockages & shields make for a nice logical package allowing the King stroll over to the mating square. Fun to watch the pieces fit together like that.
Is the problem cooked, because of the shorter sequence?
5. c1N
6. Nd3
7. Ne5
8. Nf7
9. Kf8
10. Ke8
11. Nd6
12. Nf5
13. Ne7
14. Kd8
15. Kc7
16. Nc6
17. Kb6
18. Ka5
19. Nb4
Incidentally, there is a typo. 21. Kd7 should be 21. Kc7
Never mind. Nb4 can take Qa6!